
We can continue by adding the integral to both sides:įinally, we divide by 2 and have the antiderivative we were trying to find: The integral at the end is identical to the original integral. However, if we stop on the third line we can write: The integrand on the right is the product of the last column in the row at which you stopped and the first two columns in the next row, as shown in yellow above.Īs you can see things are just repeating the lines above sometimes with minus signs. Example 2 shows why you want (need) to stop.
#TABULAR INTEGRATION BY PARTS HOW TO#
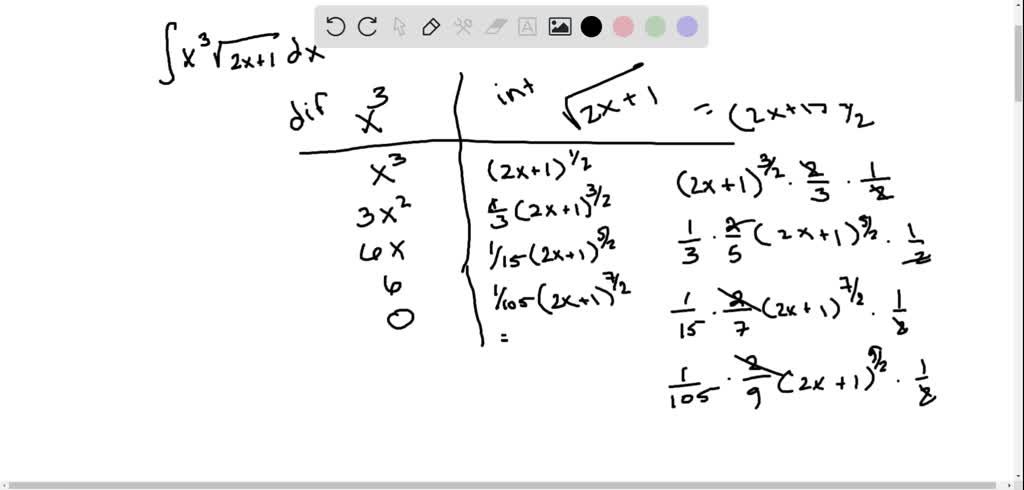
There are ways to complete the integration as shown in the examples.Įxample 1: Find by the tabular method (See Integration by Parts 2 for more detail on how to set the table up)Īdding the last column gives the antiderivative: Why stop? Because often there will be no end if you don’t stop. To complete the topic, this post will show two other things that can happen when using integration by parts and the tabular method.įirst we look at an example with a polynomial factor and learn how to stop midway through. These are shown in the examples in the posts above and Example 1 below. The tabular method works well if one of the factors in the original integrand is a polynomial eventually its derivative will be zero and you are done. There are several ways of setting up the table one is shown here and a slightly different way is in the Integration by Parts 2 post above.

Scroll down to “Antiderivatives 5: A BC topic – Integration by parts.” The tabular method is discussed starting about time 15:16. There is also a video on integration by parts here. Modified Tabular Integration presents a very quick and slick way of doing the tabular method without making a table. Integration by Parts 2 introduces the tabular method This is as far as a BC course needs to go. Integration by Parts 1 discusses the basics of the method. Here are some previous posts on integration by parts and the tabular method Since we were getting far from what is tested on the BC Calculus exams, I ended the discussion and said for those that were interested I would post more on the tabular method this blog going farther than just the basic set up. At an APSI this summer the participants and I got to discussing the “tabular method” for integration by parts. Sameen Ahmed Khan, Doing numerical calculus using Microsoft Excel, Indian Journal of Science and Technology, 9(44), pp.1–5, 2016. Singer, Formal solutions of differential equations, Journal of Symbolic Computation, 10(1), pp.59–94, 1990 (08)80037-5. Įarl Rainville, Phillip Bedient and Richard Bedient, Elementary Differential Equations, Pearson, 2014. Simmons, Differential Equations with Applications and Historical Notes, Taylor & Francis, 2016. Padmanabhan, Dawn of science-Calculus is developed in Kerala, Resonance Journal of Science Education, 17(2), pp.106–115, 2012. īurkard Polster and Marty Ross, Math Goes to the Movies, Johns Hopkins University Press, 2012. Tisdell, Tic-Tac-Toe and repeated integration by parts: Alternative pedagogical perspectives to Lima’s integral challenge, International Journal of Mathematical Education in Science and Technology, 51(3), pp.424–430, 2020.
Weir, Thomas’ Calculus, 14th Edition, Pearson, 2018.ĭavid Horowitz, Tabular Integration by Parts, College Mathematics Journal, 21(4), pp.307–311, 1990.


 0 kommentar(er)
0 kommentar(er)
